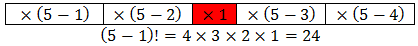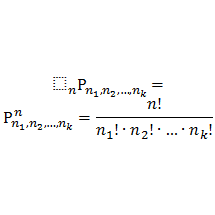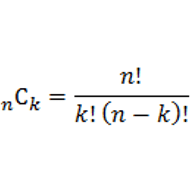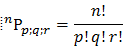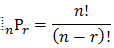The definition of permutation
Permutation is a different arrangement or a different order which is formed by a part or the whole elements which are taken from a group of elements provided. The number of permutation from k elemen which is taken from n element provides is equal to

Pay attention to the following letters arrangements. Each arrangement is consist of three some letters but have the different meaning.
{ANI,INA,NAI,NIA,AIN,IAN}
Example 7: Determine the number of arrangements or permutation of 4 letters taken from 4 letters: a, b, c, d that provided.
Answer:
The number of letters that provided is 4, which are: a, b, c, and d. Taken 4 letters, then the number of arrangements or permutation =

That 24 arrangements of letters can be investigated by tree diagram as the following.

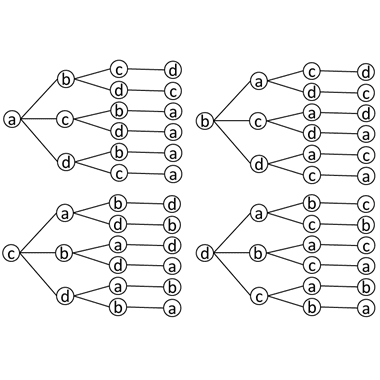
The arrangements of letters are:
abcd, abdc, acbd,
acdb, adbc, adcb,
bacd, badc, bcad,
bcda, bdac, bdca,
cabd, cadb, cbad,
cbda, cdab, cdba,
dabc, dacb, dbac,
dbca, dcab, dcba
Exercise Competency Test 7
1. The number of arrangements or permutation of 3 letters which is taken from 3 letters: a, b, c that provided, is equal to …
A. 27 B. 9 C. 6 D. 3 E. 1
Answer: C, explanation:
{abc, acb, bac, bca, cab, cba}.

2. Four official are invited, they come not at the same time. The number of ways of their arrival is equal to …
A. 1 B. 4 C. 8 D. 24 E. 48
Answer: D, explanation:

3. A shop will sell 5 kinds of television. The number of ways to make the price lists is equal to …
A. 120 B. 60 C. 30 D. 15 E. 5
Answer: A, explanation:

5!=₅P₅=5×4×3×2×1=120

4. Five stickers will be stuck to a line at five places provided. If one of that 5 stickers is always on the middle, then the number of ways to stick the stickers is …
A. 120 B. 60 C. 24 D. 20 E. 12
Answer: C, explanation: